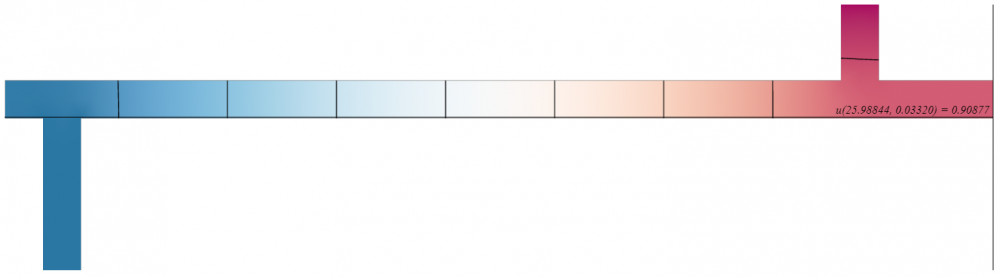-
Eigenranking
My team matrix: [
[0, 1, 1, 3, 1],
[2, 0, 2, 2, 2],
[3, 4, 0, 1, 4],
[1, 4, 1, 0, 2],
[4, 3, 2, 3, 0]
]
The ranking for the league was:
Team 3: rating = 0.5616064344284055 Team 5: rating = 0.5279808500356232 Team 2: rating = 0.4002177984108782 Team 4: rating = 0.3946242396754421 Team 1: rating = 0.29988640866168415
Team 3 was the best.
-
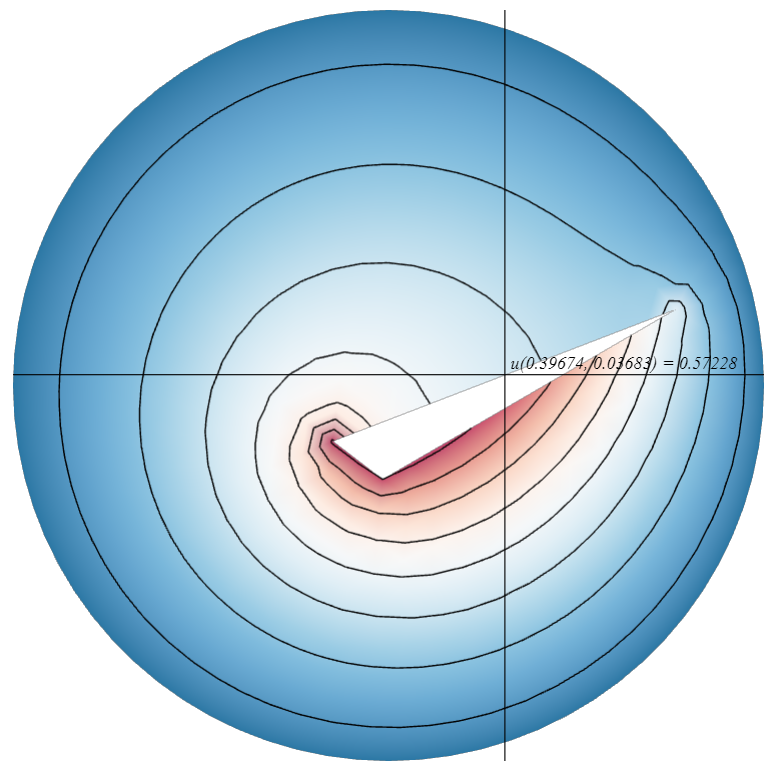
Modeling a steady state heat distribution in 2D
-
Modeling 2D Heat Flow
-
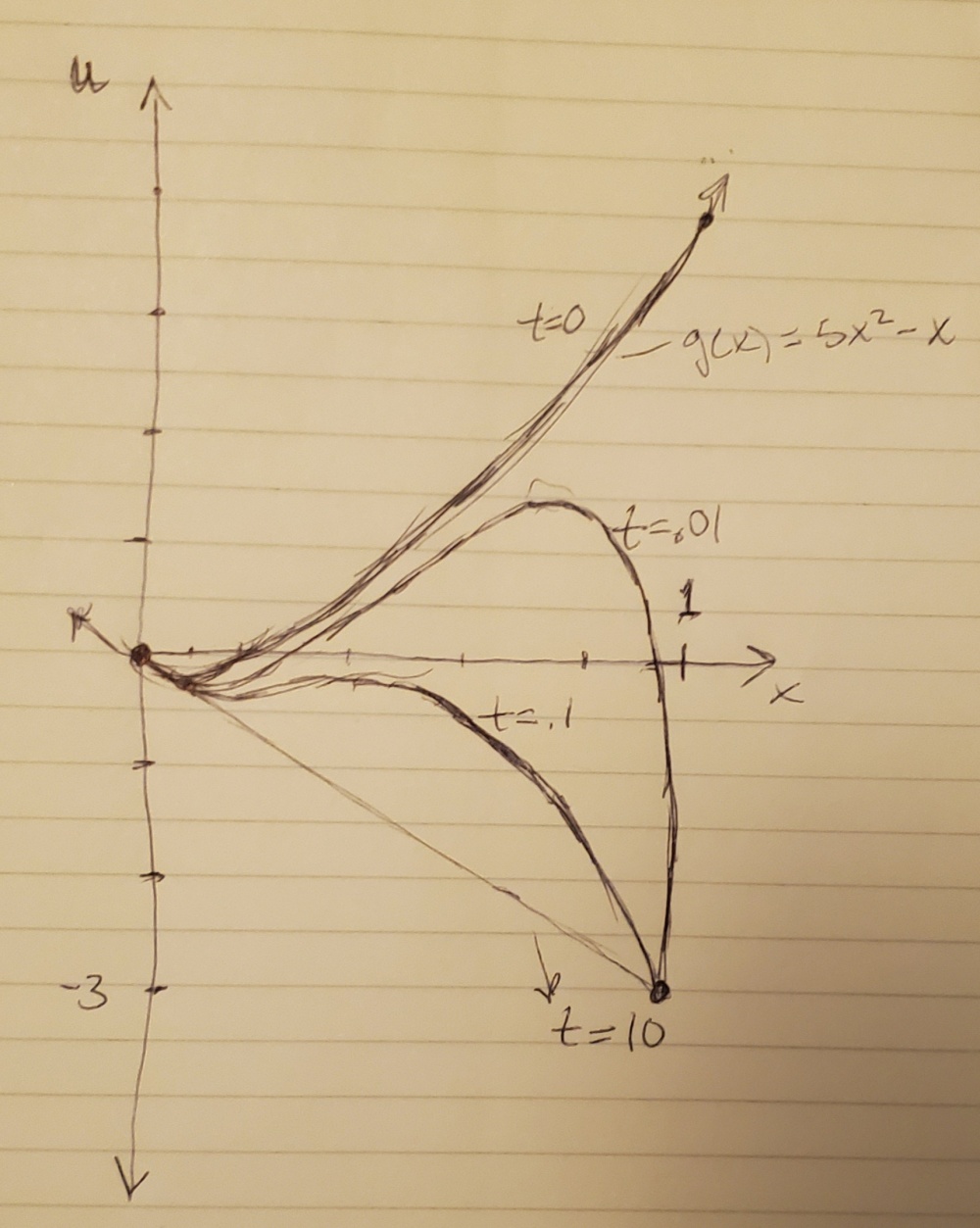
Random heat evolution problem
-
Steady state heat flow with source
The heat flow equation with a constant internal heat source I was given is
$$u_t=u_{xx}+5.$$
My boundary conditions are
$$u(0,t)=-4\mbox{ and }u(1,t)=5.$$
To find the steady state temperature distribution we set the partial derivative of the function $u(x,t)$ with respect to time equal to zero, that is $u_t=0$, and then integrate in succession, yielding
$$u(x,t)=-\frac{5}{2}x^2+c_1x+c_2.$$
Using the given boundary conditions, $u(0,t)=-4$, $u(1,t)=5$, we determine $c_1=\frac{23}{2}$ and $c_2=-4$. Thus the steady state temperature distribution is represented by
$$u(x,t)=-\frac{5}{2}x^2+\frac{23}{2}x-4.$$