Subsection 1.2.1 The iteration of real functions
We were introduced to the idea of real iteration back in Subsection 1.1.2 but there's no reason the function we iterate needs to come from Newton's method. Figure 1.17 illustrates iteration using a geometric cool called a cobweb plot. In part (a) we see the iteration of \(N(x)=x/2+1/x\text{,}\) that arises in Newton's method. In part (b) we the iteration of \(f(x)=3.1x(1-x)\text{.}\) This is an example of a logistic function and really has nothing directly to do with Newton's method.
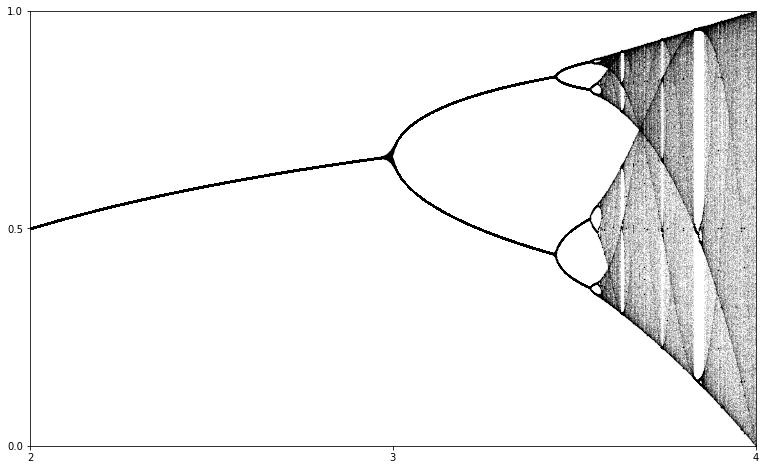
The logistic function above is a member of a family of functions - the logistic family. These functions have the form \(f_r(x) = rx(1-x)\text{.}\) When studying a family of functions like this we are often interested in the various types of behavior that might arise. We can explore these possibilites with a tool called a bifurcation diagram. The bifurcation diagram for the logistic family is shown in Figure 1.18.